The Glass Transition in Polymers (all content)
Note: DoITPoMS Teaching and Learning Packages are intended to be used interactively at a computer! This print-friendly version of the TLP is provided for convenience, but does not display all the content of the TLP. For example, any video clips and answers to questions are missing. The formatting (page breaks, etc) of the printed version is unpredictable and highly dependent on your browser.
Contents
Main pages
Additional pages
Aims
On completion of this TLP you should:
- understand what is meant by the glass transition in amorphous polymers, and its causes
- understand kinetic and thermodynamic analyses of the formation of glasses
- appreciate that the value of the glass transition temperature depends on the method of measurement, and also on the strain rate or cooling or heating rate
- understand how the glass transition affects the properties of rubber
Introduction
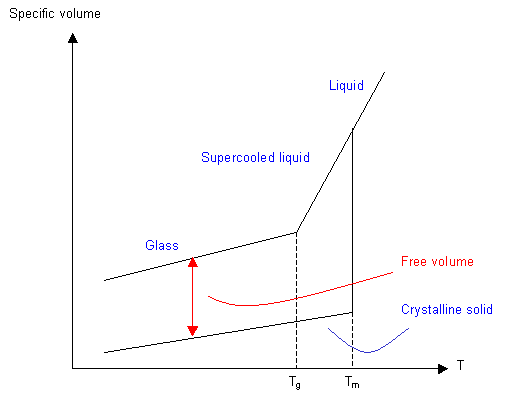
Collections of molecules can exist in three possible physical states: solid, liquid and gas. In polymeric materials, things are not so straightforward. For example most polymers will decompose before they boil, and cross-linked polymers decompose before they melt. For many polymers the transition between the solid and liquid states is rather diffuse and difficult to pinpoint. Amorphous polymers are viscous liquids when they are held at temperatures above their glass transition temperature, Tg. Below Tg, the material is solid, yet has no long-range molecular order and so is non-crystalline. In other words, the material is an amorphous solid, or a glass.
The glass transition temperature is different for each polymer, but many polymers are above Tg at room temperature. In many cases the polymers are at least partially crystalline at room temperature and the temperature at which the crystals melt (Tm) is above room temperature. The graph below shows how some polymers are above Tg but below Tm at room temperature. Such polymers are rubbers (so long as they are largely amorphous) at room temperature. However, the polymer may flow like a liquid over long time periods as its amorphous component relaxes under the polymer's weight.
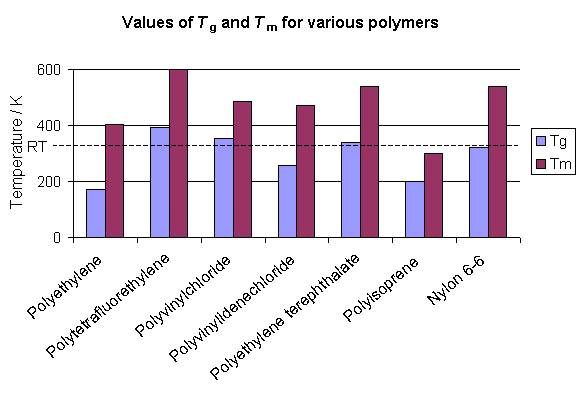
The glass transition of a polymer is related to the thermal energy required to allow changes in the conformation of the molecules at a microscopic level, and above Tg there is sufficient thermal energy for these changes to occur. However, the transition is not a sharp one, nor is it thermodynamically well defined. It is therefore different from melting a crystal, as will be explained later.
A distinct change from rubbery (above Tg) to glassy (below Tg) behaviour is readily observable in a wide range of polymers over a relatively narrow temperature range. In the following sections the behaviour of polymers around the glass transition temperature will be explored. The effects of strain rate, cooling or heating rate and other factors affecting the glass transition temperature will also be explained.
Theory 1
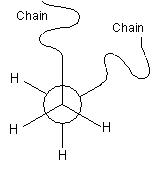
When we extend or compress a polymer elastically (i.e. there is no permanent deformation), we try to move the chain-ends apart or together. For a simple polymer chain to change its conformation, individual C-C bonds must twist from the trans to gauche position or vice versa - i.e. the torsion angles must change. This is a thermally activated process. At low temperatures, there is not enough thermal energy available to allow torsion angle changes, so the conformation becomes frozen in. The temperature above which the torsion angles can change is called the glass transition temperature. The changes in conformation also depend on time-scale, so the apparent value of Tg depends on the time-scale over which the behaviour is being monitored.
The strain in a polymer is accommodated by the change in shape of the individual molecules, but it should be noted that the response of the bulk polymer is influenced by the interactions between the molecules. This affects the ability of the bonds to rotate, and also the viscosity of the bulk polymer.
Therefore Tg depends on the polymer's architecture, and there are several factors influencing the transition:
Chain Length
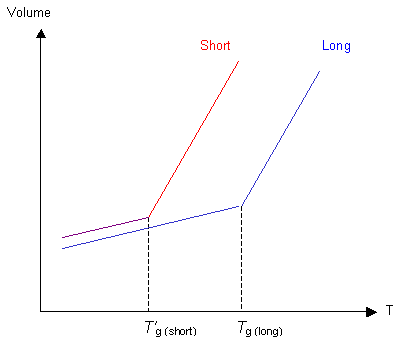
Each chain end has some free volume associated with it. A polymer with shorter chains will have more chain ends per unit volume, so there will be more free volume. Hence Tg' for shorter chains will be lower than Tg for long chains. Note that the shorter-chained polymer also has more free volume frozen in below Tg than the long-chained polymer.
Chain Flexibility
A polymer with a backbone that exhibits higher flexibility will have a lower Tg. This is because the activation energy for conformational changes is lower. Therefore, conformational changes can take place at lower temperatures.
Side Groups
Larger side groups can hinder bond rotation more than smaller ones, and therefore cause an increase in Tg. Polar groups such as Cl, CN or OH have the strongest effect.
Branching
Polymers with more branching have more chain ends, so have more free volume, which reduces Tg, but the branches also hinder rotation, like large side groups, which increases Tg. Which of these effects is greater depends on the polymer in question, but Tg may rise or fall.
Cross-linking
Cross-linking reduces chain mobility, so Tg will be increased. It also affects the macroscopic viscosity of the polymer, since if there are cross-links between the chains, then they are fixed relative to each other, so will not be able to slide past each other.
Plasticisers
Small molecules, typically esters, added to the polymer increase the chain mobility by spacing out the chains, and so reduce Tg.
Time Effects
The properties of an amorphous polymer above Tg can change with time
- At very short loading times the polymer can still be glassy because there is not time for the chains to move.
- At intermediate times the polymer may be rubbery - i.e. chains can uncoil and recoil between entanglements, which remain stable.
- At very long times, the chains can move past each other permanently, and so the polymer behaves as a viscous liquid.
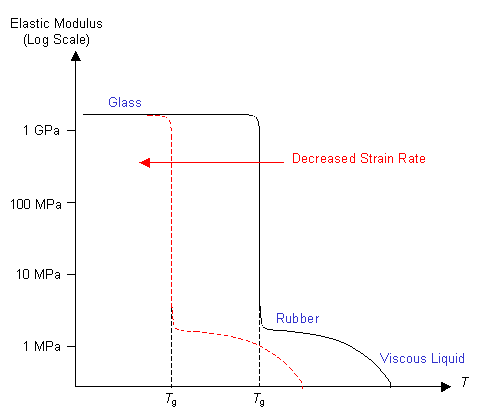
For a useful rubbery material it is necessary to suppress chain sliding. One way of doing this is to increase the amount of cross-linking in the polymer. In order for the chains to slide the cross-linking bonds must first be broken. So increasing the number of cross-links decreases the chains' ability to slide over each other. This extends the rubbery region at higher temperatures, so the graph looks like this:
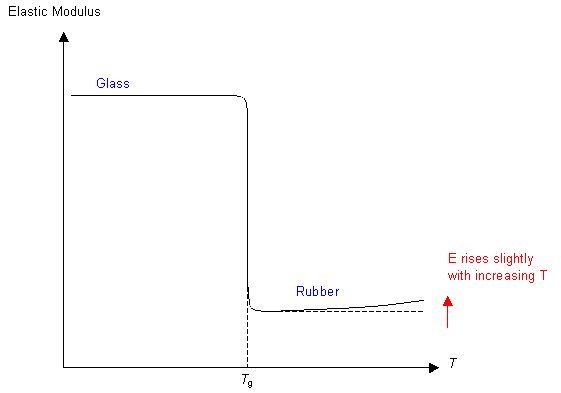
More about the slight rise in elastic modulus with increasing temperature in rubber is available in the Stiffness of Rubber TLP.
Theory 2 - kinetics vs thermodynamics
The formation of glasses can be understood from both a kinetic approach and a thermodynamic approach. In this section we will review both.
Kinetic Approach to Glasses
Consider a polymeric liquid being cooled towards its melting temperature. Once the temperature of the liquid reaches Tm the solid crystalline phase is thermodynamically favourable. In order for the liquid to undergo a phase transition to the solid state a two-step process must take place:
- Nucleation of solid seeds
- Growth of the seeds
Nucleation is the formation of small crystalline solid particles in the liquid. As a result a new interface is formed in between the solid particle and the liquid. This interface has an associated energy, the interfacial energy. For a successful nucleation event to occur the polymer must find extra energy from somewhere to "pay" for the energy cost of creating a new interface.
This energy is called the "driving force" for nucleation. As we cool the liquid just below Tm we see no nucleation at first. Instead nucleation occurs at a temperature T< Tm, below the expected temperature. When the polymer is still liquid below Tm it is said to be undercooled. The amount of "undercooling" is limited by the nucleation rate.
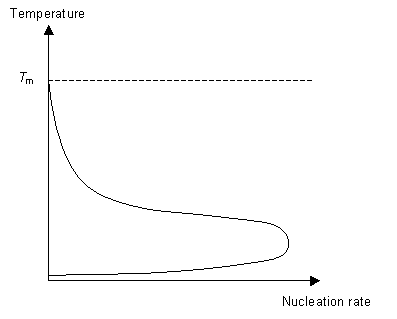
The second stage of the phase transition is the growth of the nucleated seeds. This is a thermally activated process, which means that its rate is dependent on the temperature. So for fast crystal growth the ideal method is to cool the polymer to just above Tg to allow for nucleation to occur and then raise the temperature to just below Tm to allow growth to occur.
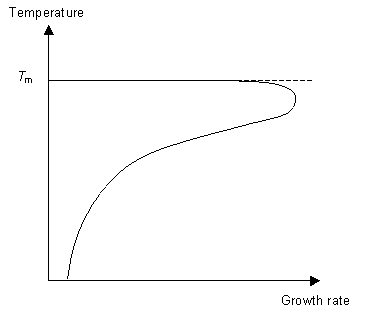
Understanding these concepts we can now think about how best to cool a liquid in order to form a glass. To ensure we form a glass we want to reduce the number of nucleation events as much as possible (otherwise we will have crystals not an amorphous solid) and not allow growth to occur. By cooling the liquid very quickly (i.e. quenching it) it is possible to reduce the mobility of the molecules to the point where they can not move around to order themselves periodically as they do not have the energy to diffuse far enough. This concept can be expressed on a TTT (time temperature transformation) graph.
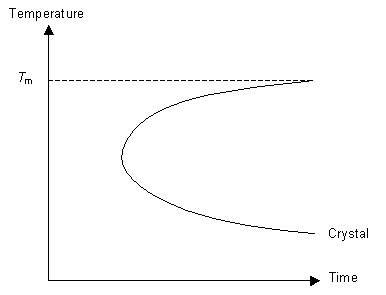
The graph represents the degree of crystallinity of the polymer as it is cooled. The line marked "crystal" indicates a specific degree of crystallinity (for example it may indicate 90% crystallinity in the sample). The TTT graph is used for isothermal transformations, but here it is loosely applied to continuous cooling.
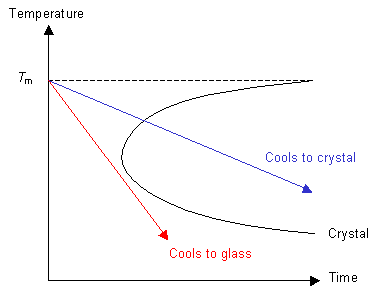
If the cooling rate is fast enough then the polymer can be cooled so that it does not enter the crystal region of the graph. In this case, although the polymer is below Tm, it does not crystallise because the molecules can not move to order themselves periodically (as in a crystal). Instead the polymer has formed an amorphous solid, or a glass.
Thermodynamic Approach to Glasses
Consider the red line from the graph above. Above Tm the polymer is a liquid. At temperatures a long way below Tm the polymer behaves like a solid, although it is not crystalline. In this state the glass has the properties of a solid, but may exhibit aspects of liquid behaviour over long time scales.
(There is a common misconception that inorganic glasses behave in this same way. This liquid behaviour is incorrectly thought to be responsible for the windows of old buildings often being thicker at the bottom than at the top. This is discussed briefly, with citations, for those who are interested on a separate page in this TLP.)
Have a look at the graph of the polymer's enthalpy at different temperatures:
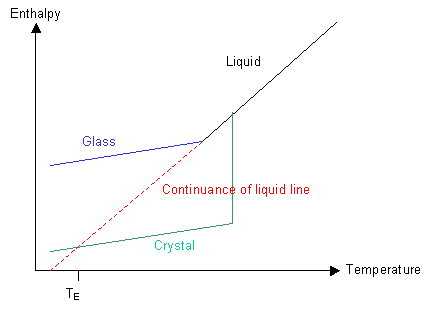
If nucleation can occur and the rate of cooling is not too high, then the green line will be followed and the polymer will crystallise below Tm to form a crystalline solid.
If the polymer is quenched so that ordering of the molecules cannot take place then the blue line will be followed and the polymer will form a glass below Tm.
Let us now imagine what would happen if we could follow the red line and keep cooling the liquid without forming a crystalline solid. There is a point, TE, at which the enthalpy of the supercooled liquid falls below that of the crystalline solid. Similarly, there is a temperature, TK, the Kauzmann temperature, below which the entropy of the liquid would be less than that of the corresponding solid. In other words, below the Kauzmann temperature the liquid should be more ordered than the corresponding solid. As we know, solids are highly ordered and liquids are not, so the liquid cannot reach this condition.
The paradox is avoided because by the time the Kauzmann temperature has been reached on cooling, the liquid has gone through transition into a glassy state.
Important points relating to phase transitions within polymers
- Glass formation can be explained both kinetically and thermodynamically
- Glasses can be formed by quenching (rapidly cooling) the polymer so that the molecules do not have time to order themselves into a periodic crystal
- Glasses exhibit the properties of a solid, but over long time scales can flow like a liquid
- Below the Kauzmann temperature the polymer must either be a crystalline solid or a glass as the liquid phase is thermodynamically unstable
Measurement of Tg
There are several methods available to measure the glass transition temperature, some of which are given below. Since the value of the glass transition temperature depends on the strain rate and cooling or heating rate, there cannot be an exact value for Tg.
Mechanical Methods
It is possible to calculate a value for the glass transition temperature by measuring the elastic modulus of the polymer as a function of the temperature, for example by using a torsion pendulum. Around Tg there is a large fall in the value of the modulus. The frequency of the oscillation is important, since Tg depends on the time allowed for chain segment rotation.
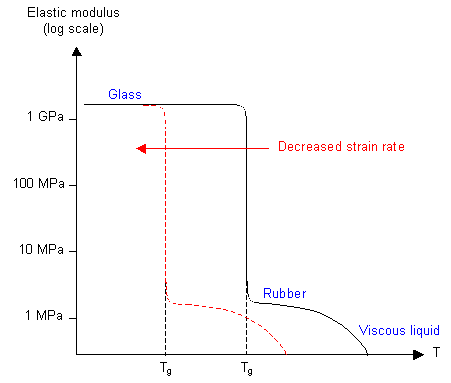
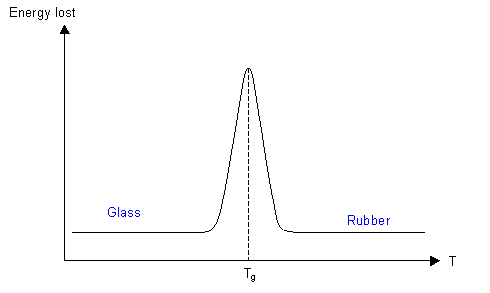
A more common method is dynamic mechanical thermal analysis (DMTA), which measures the energy absorbed when a specimen is deformed cyclically as a function of the temperature, and a plot of energy loss per cycle as a function of temperature shows a maximum at Tg.
Thermal Methods
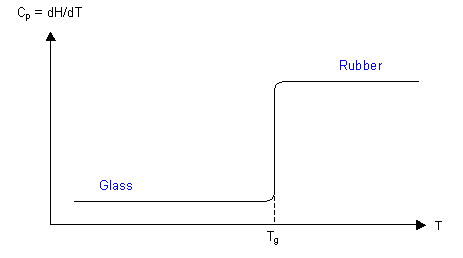
As was shown in the thermodynamic approach to glasses, the enthalpy of a polymer decreases as the temperature decreases, but with a change in slope in the graph at Tg. Taking the derivative of this graph with respect to temperature, the specific heat capacity can be plotted, as below. The specific heat capacity, Cp, can be measured using calorimetry, e.g. differential scanning calorimetry (DSC). The value of Tg depends on the heating or cooling rate.
Volume Methods
The changes in conformation that occur above Tg require more volume, so plotting a graph of specific volume or thermal expansion coefficient against temperature will give a value for Tg. The actual volume of the molecules stays the same through Tg, but the free volume (the volume through which they can move) increases.
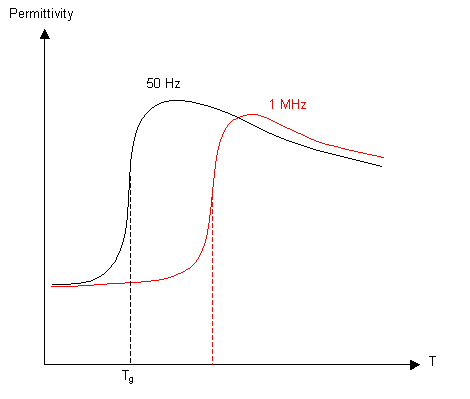
Dielectric Constant
If a varying electric field is applied to a polymeric material, any polar groups will align with the field. Below Tg rotation of the bonds is not possible, so the permittivity will be low, with a big increase around Tg. At higher temperatures the increased thermal vibrations cause the permittivity to drop again. If the frequency of the field is increased, the polar groups have less time to align, so the glass transition occurs at a higher temperature.
Demonstrations
Bouncy Balls
A bouncy ball is made of a polymer that is above its Tg at room temperature, with cross-linking keeping the ball in its spherical shape. The height that the ball rebounds to when dropped is dependent on how much energy is lost during the bounce. At room temperature the ball loses little energy when it deforms, so can rebound to a large fraction of its original height. As the temperature is reduced, the viscosity of the polymer increases, so more of the elastic strain energy is dissipated, and the ball does not bounce as high.

At Tg nearly all the energy is dissipated, and the ball barely bounces at all. As the temperature is reduced more to below Tg, there is not enough energy for conformational changes to occur, and the ball becomes glassy. The energy losses above Tg are due to the viscosity as the conformation changes and the polymer chains move past each other, and since these movements do not occur, the energy is not dissipated, so the ball bounces again.
The videos show how high a bouncy ball bounces at different temperatures. The videos were filmed at 500 frames a second, and played back at 50 frames a second, so are at one tenth of actual speed.
Ball bouncing at 25°C, well above Tg |
Ball bouncing at -50°C, just above Tg |
Ball bouncing at -70°C, close to Tg |
Ball bouncing at -190°C, well below Tg |
The second set of videos are close ups of the bounce itself, showing the deformation of the ball. The ball bounces both above and below Tg, although the process is very different. Above Tg the conformation of the polymer chains changes, and the deformation of the ball can clearly be seen. Below Tg, the conformation is frozen, so it is only the interatomic bonds that are strained, and the macroscopic deformation is much less, and is not observable in the videos. bouncing
Rubbery bounce, well above Tg |
Bounce near Tg |
|
Glassy bounce, well below Tg | |
Silly Putty
The time dependence on the deformation of a material that exhibits a glass transition can be demonstrated with "Silly Putty". If a ball of putty is left at rest at room temperature, then it will slowly deform plastically under its own weight. However, if the ball of putty is hit with a hammer, again at room temperature, then it is possible to smash the putty into pieces.
Silly putty deforming under its own weight |
Silly putty striking the ground |
|
Silly putty being hit by a hammer | |
The first video shows a small ball of putty (initially approximately 40 mm diameter) deform under its own weight at room temperature. The deformation took just over 40 minutes, so the film has been speeded-up 80 times. The height of the ball can be judged against the background. The initial deformation is much more rapid than towards the end, as the following numbers show:
| Loss of height / mm | Time / mins:secs |
| 5 | 1:10 |
| 10 | 5:30 |
| 15 | 25:00 |
| 16 | 42:00 |
The other two videos were both filmed at 1000 frames per second, and played back at 4 frames per second, so are at 1/250th of real speed.
The second video is of a ball of putty hitting the ground after being dropped from a height of 1 m. The ball deforms, but returns to its original shape as it rebounds. When a ball of putty is dropped it rebounds to a large fraction of its original height.
The third video is of a ball of putty being hit with a hammer, also at room temperature. The theory states that at fast strain rates the material will be glassy, and this is shown in this video, although it may not seem like it at first. The ball deforms quite a lot initially, and then breaks apart. This can be explained by the putty being glassy, with an elastic modulus low enough for there to be a visible strain before a critical strain is reached and brittle fracture occurs.
Summary
You should now be familiar with the following concepts:
-
Above Tg polymers are rubbery, whereas below Tg they are glassy
-
Rubbery behaviour arises from the polymer's ability to change its conformation at high temperatures
-
Glassy behaviour arises from the polymer's lack of ability to change its conformation at low temperatures
-
Many factors affect the Tg of a polymer. Some factors are to do with the chemistry of the polymer:
- chain length
- chain flexibility
- side groups
- branching
- cross-linking
- the presence of plasticisers
Other factors are to do with the method of measuring Tg:
- strain rate
- cooling or heating rate
-
You should also understand the theory of kinetics, which explains how glasses form and noted that there are many different experimental methods for measuring the glass transition temperature in polymers.
-
Finally you should have observed how the behaviour of a rubber ball varies when it is bounced above, below and near its Tg and seen how the behaviour of silly putty varies at different strain rates.
Questions
-
Explain why cooling a liquid very fast (quenching) can lead to the formation of a glass. Your answer should use what you have learnt about the kinetics of glass formation.
-
What is meant by the 'free volume' of a polymer?
-
What is the significance of the theoretical 'Kauzmann temperature'?
-
Why is the measured value of Tg dependent on the method of measurement?
-
Using what you understand about the way polymer chains behave above and below Tg, why do you think glasses tend to be brittle whereas rubbers are not?
-
What is meant by the conformation of a polymer? Using a Newman projection show what is meant by the 'trans' and 'gauche' states.
-
Explain how the dielectric constant of a polymer is affected by the transition to the glassy state.
-
Explain why the glass transition temperature is strain-rate dependent.
-
Below and above the Tg of a rubber ball it will rebound after impact. At Tg the ball will not rebound very much at all. Explain how the ball bounces in these three cases from an energetic point of view.
Going further
Websites
- The Glass Transition
Part of the Macrogalleria based at the Department of Polymer Science at the University of Southern Mississippi. - Crazy Aaron's Puttyworld
Includes video of a huge ball of silly putty being dropped from a tall building!
Conformation
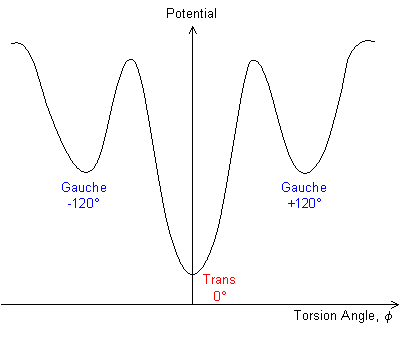
The conformation of a polymer molecule is the arrangement of the bond orientations, both within the backbone, and any side-groups and branches. There are three specific orientations, called trans, +gauche and -gauche, as represented in the Newman projections below. The conformation can be changed by rotation about single bonds.
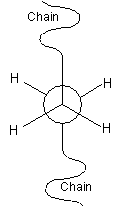 |
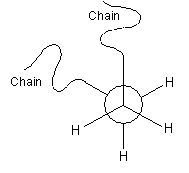 |
 |
|
Trans |
+ Gauche |
- Gauche |
The angle of rotation away from the trans-conformation is called the torsion angle, f, and the free energy of the bond in question can be plotted as a function of this angle.
Free volume
A small amount of unfilled volume is associated with the end of a polymer chain. This volume is called the free volume and is schematically represented in the diagram below.

For a given mass of polymer the amount of free volume will depend on the number of chain ends, hence the number of chains and hence the degree of polymerisation.
Do Cathedral Glasses Flow?
 A popular world-wide myth about inorganic glasses based on silica frameworks, such as glass windows in cathedrals, is that over hundreds of years noticeable flow is able to occur under the influence of gravity, as a result of which cathedral glass windows are thicker at the bottom than the top.
A popular world-wide myth about inorganic glasses based on silica frameworks, such as glass windows in cathedrals, is that over hundreds of years noticeable flow is able to occur under the influence of gravity, as a result of which cathedral glass windows are thicker at the bottom than the top.
There are a number of useful references in the scientific literature where this is discussed at some length. In a letter entitled ‘Fact or fiction? Can cold glass flow under its own weight and what happens to stained glass windows?’ sent to the Editor of Glass Technology, 37, 143 (1996) R.G. Newton notes that at room temperature inorganic glass can flow if a large enough compressive stress is applied, so that for example hardness indents can be made in inorganic glass. However, irreversible flow can only occur if stresses of at least one tenth of the theoretical breaking strength of glass are applied. Such stresses are not achievable in inorganic glass because of the presence of surface defects, which will cause the glass to shatter well before such a stress can be applied.
Further discussions of this myth, with calculations to show conclusively why cathedral glass will not flow under its own weight over hundreds of years, are to be found in:
- F.W. Preston, ‘The post-instantaneous creep of long-loaded glass specimens’, Glass Technology, 14, 20-30 (1973)
- E.D. Zanotto, ‘Do cathedral glasses flow?’, American Journal of Physics, 66, 392-395 (1998)
- E.D. Zanotto and P.K. Gupta, ‘Do cathedral glasses flow?-Additional remarks’, American Journal of Physics, 67, 260-262 (1999)
A telling point made by Zanotto and Gupta from their calculations is that the timescale over which flow might be seen at room temperature (termed the relaxation time) of 1023 years is well beyond the age of the Universe (1010 years). The explanation for why cathedral glasses are thicker at their bottom than at their tops lies in the manufacture of ancient glass. Such glass was made by blowing the glass into large cylinders that were split and flattened manually. This manufacturing process produced glass that was inherently not of a uniform thickness, unlike the glass made by the float glass process that we have today.
Instinct would make fitters of window panes in cathedrals put thicker sides of glass at the bottom, little realising that their actions would give rise to the urban myth that would sweep the globe hundreds of years later.
** Image from http://commons.wikimedia.org/wiki/File:Peterborough_Cathedral_glass_05.JPG
Academic consultant: Lindsay Greer (University of Cambridge)
Content development: Simon Adelman, Mark Wharton
Photography and video: Brian Barber, Carol Best and Ruth Hammond
Web development: Dave Hudson
DoITPoMS would like to thank the PCS Fracture and Shock Physics Group in the Cavendish Laboratory for the use of their high speed photography facilities.
This TLP was prepared when DoITPoMS was funded by the Higher Education Funding Council for England (HEFCE) and the Department for Employment and Learning (DEL) under the Fund for the Development of Teaching and Learning (FDTL).
Additional support for the development of this TLP came from the Armourers and Brasiers' Company and Alcan.

