Phase Diagrams and Solidification (all content)

Note: DoITPoMS Teaching and Learning Packages are intended to be used interactively at a computer! This print-friendly version of the TLP is provided for convenience, but does not display all the content of the TLP. For example, any video clips and answers to questions are missing. The formatting (page breaks, etc) of the printed version is unpredictable and highly dependent on your browser.

Contents

Main pages

Additional pages

Aims

On completion of this TLP you should:

• understand the thermodynamic principles behind free-energy curves
• understand how free-energy curves relate to equilibrium phase diagrams
• be able to construct a binary phase diagram from cooling curves
• be able to use phase diagrams to predict the composition and volume fraction of phases

Introduction

The phase diagram is a crucial part of metallurgy - it shows the equilibrium states of a mixture, so that given a temperature and composition, it is possible to calculate which phases will be formed, and in what quantities. As such it is very valuable to be able to construct a phase diagram and know how to use it to predict behaviour of materials.

The main theory behind phase diagrams is based around the latent heat that is evolved when a mixture is cooled, and changes phase. This means that by plotting graphs of temperature against time for a variety of different compositions, it should be possible to see at what temperatures the different phases form.

It is relatively easy to produce a rough binary phase diagram, as will be shown later in the package, but although it is quick to take readings for the top part of a phase diagram, it takes longer, and hence more sensitive equipment to monitor the changes that take place when a solid changes phase. A typical simple binary phase diagram is as follows:

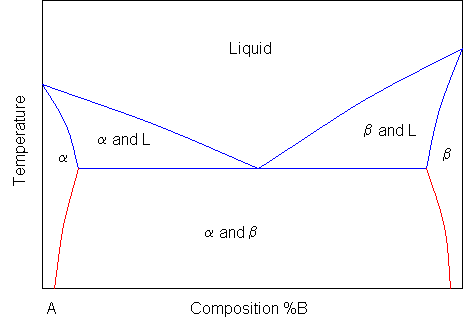
Where L stands for liquid, and A and B are the two components and α and β are two solid phases rich in A and B respectively. The blue lines represent the liquidus and solidus lines, which are relatively simple to measure. The red lines involve a solid-to-solid transition, and so require much more sensitive equipment.

However, there is also a lot of thermodynamic theory behind phase diagrams, which allows more problematic or more complex systems to be predicted, and this can lead to faster creation of phase diagrams, as it can take a long time to pick up all the stable phases in experiments, and there is not always the time available for such practical work.

A crucial point to remember is that a phase diagram should always display the equilibrium phases, and so with cooler temperatures, these are hard to attain due to kinetic problems. Even at higher temperatures, there may be problems of having enough time for the solid to fully equilibrate as the system is cooling.

Thermodynamics: Basic terms

Internal Energy, U

The internal energy of a system is the sum of the potential energy and the kinetic energy. For many applications it is necessary to consider a small change in the internal energy, dU, of a system.

dU = dq + dw = CdT - PdV

dq = the heat supplied to a system
dw = the work performed on the system
C = heat capacity
dT = change in temperature
P = pressure
dV = change in volume

At constant volume,

dU = C_VdT

Enthalpy, H

Enthalpy is the constant pressure version of the internal energy. Enthalpy,

H = U + PV.

Therefore, for small changes in enthalpy,

dH = dU + PdV + VdP.

At constant pressure,

dH = C_PdT.

Entropy, S

Entropy is a measure of the disorder of a system. In terms of molecular disorder, the entropy consists of the configurational disorder (the arrangement of different atoms over identical sites) and the thermal vibrations of the atoms about their mean positions. A change in entropy is defined as,

\[dS \ge \frac{{{\rm{d}}q}}{T}\]

For reversible changes, i.e. changes under equilibrium conditions,

dq = TdS

For natural changes, i.e. under non-equilibrium conditions,

dq < TdS

Gibbs free energy, G

The Gibbs free energy can be used to define the equilibrium state of a system. It considers only the properties of the system and not the properties of its surroundings. It can be thought of as the energy which is available in the system to do useful work.

Free energy, G, is defined as,

G = H - TS = U + PV - TS

For small changes,

dG = dH - TdS - SdT = VdP - SdT + (dq - TdS)

For changes occurring at constant pressure and temperature,

dG = dq - TdS

Therefore, dG = 0 for reversible (equilibrium) changes, and dG < 0 for non-reversible changes.

From this it is clear that G tends to a minimum at equilibrium.

The Helmholtz free energy, F, is sometimes used instead of G, and is the equivalent of G for changes at constant volume. It is defined as,

F = U - TS

Thermodynamics of Solutions

Consider a mechanical mixture of two phases, A and B. If this is then transformed into a single solution phase with A and B atoms distributed randomly over the atomic sites, then there will be,

• An enthalpy change associated with interactions between the A and B atoms, ΔH_mix
• An entropy change, ΔS_mix, associated with the random mixing of the atoms
• A free energy of mixing, ΔG_mix = ΔH_mix - TΔS_mix

Assume that the system consists of N atoms: x_AN of A and x_BN of B, where,

x_A = fraction of A atoms and x_B = (1 - x_A) = fraction of B atoms

Enthalpy of mixing

In calculating ΔH_mix it is assumed that only the potential energy term undergoes any significant change during mixing. This change arises from the interactions between nearest-neighbour atoms. Consider an alloy consisting of atoms A and B. If the atoms prefer like neighbours, A atoms will tend to cluster and likewise B atoms, so a greater number of A-A and B-B bonds will form. If the atoms prefer unlike neighbours a greater number of A-B bonds will form. If there is no preference A and B atoms will be randomly distributed.

Let w_AA be the interaction energy between A - A nearest neighbours, w_BB that for B - B nearest neighbours and w_AB that for A - B nearest neighbours.

All of these energies are negative, as the zero in potential energy is for infinite separation between atoms.

Let each atom of A and B have co-ordination number z.

Therefore, the total number of nearest-neighbour pairs is Nz/2.

Probability of A - A neighbours = x_A²

Probability of B - B neighbours = x_B²

Probability of A - B neighbours = 2x_Ax_B

For a solid solution the total interaction energy is,

H_s - U_s = Nz/2 (x_A² w_AA + x_B² w_BB + 2x_Ax_B w_AB)

For pure A, H_A = (Nz/2)w_AA

For pure B, H_B = (Nz/2)w_BB

Hence the enthalpy of mixing is given by,

ΔH_mix = H_s - (x_AH_A + x_BH_B) = (Nz/2)x_Ax_B (2w_AB - w_AA - w_BB)

We can define an interaction parameter

W = (Nz/2)(2w_AB - w_AA - w_BB)

Therefore,

ΔH_mix = Wx_Ax_B

If A-A and B-B interactions are energetically more favourable than A-B interactions then W > 0. So, ΔH_mix > 0 and there is a tendency for the solution to form A-rich and B-rich regions.

If A-B interactions are energetically more favourable than A-A and B-B interactions, W < 0, ΔH_mix < 0, and there is a tendency to form ordered structures or intermediate compounds.

Finally if the solution is ideal and all interactions are energetically equivalent, then W = 0 and ΔH_mix = 0.

Entropy of mixing

Per mole of sites, this is

ΔS_mix = kN (- x_Alnx_A - x_Blnx_B)

(the derivation of this result makes use of Stirling's approximation)

where N = Avogadro's number, and kN = R, the gas constant.

Hence,

ΔS_mix = R (- x_Alnx_A - x_Blnx_B)

A graph of ΔS_mix versus x_A has a different form from ΔH_mix. The curve has an infinite gradient at x_A = 0 and x_A = 1.

The free energy of mixing is now given by,

ΔG_mix = ΔH_mix - TΔS_mix = x_Ax_BW + RT (x_A lnx_A + x_Blnx_B)

For W < 0, ΔG_mix is negative at all temperatures, and mixing is exothermic. For W > 0, ΔH_mix is positive and mixing is endothermic.

Free energy curves

For any phase the free energy, G, is dependent on the temperature, pressure and composition.

Pure Substances

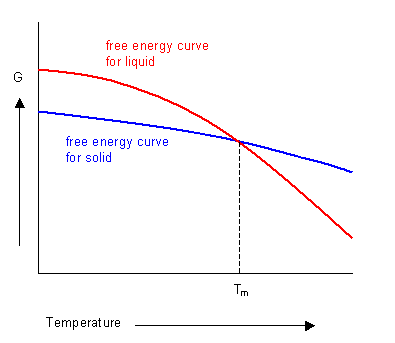
For pure substances the composition does not vary and there is little dependence on pressure. Therefore the free energy varies greatest with temperature.

The phase with the lowest free energy at a given temperature will be the most stable. The curves for the free energies of the liquid and solid phases of a substance have been plotted below. It shows that below the melting temperature the solid phase is most stable, and above this temperature the liquid phase is stable. At the melting temperature, where the two curves cross, the solid and liquid phases are in equilibrium.

Solutions

Solutions contain more than one component and in these situations the free energy of the solution will become dependent on its composition as well as the temperature.

It is shown above that the free energy of mixing is:

ΔG_mix = ΔH_mix - TΔS_mix = x_Ax_BW + RT (x_A lnx_A + x_Blnx_B)

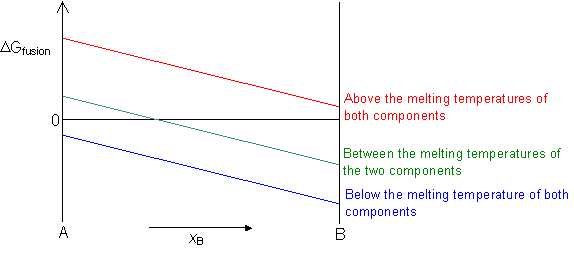
The shape of the ΔG_mix curve is dependent on temperature . For the curve shown below the value of ΔH_mix is positive, leading to a maximum on the curve at low temperatures. ΔG_mix is always negative for low solute concentrations as the gradient of ΔS_mix is infinite at x_A = 0 and x_A = 1.

At high temperatures there is a complete solution and the curve has a single minimum. At low temperatures the curve has a maximum and two minima. In the composition range between the two minima (denoted by the dashed lines) a mixture of two phases is more stable than a single-phase solution.

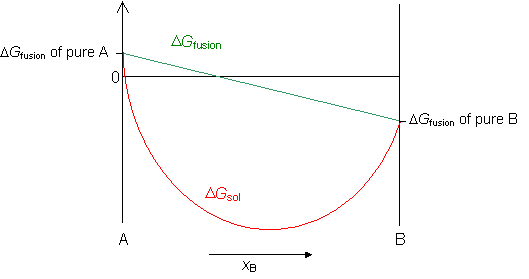
The free energy of a regular solid solution, ΔG_sol, is the sum of the free energy of mixing ΔG_mix and the free energy of fusion ΔG_fus.

Free energy of fusion

When a liquid solidifies there is a change in the free energy of freezing, as the atoms move closer together and form a crystalline solid. For a pure component, this can be empirically calculated using Richard's Rule:

ΔG_fusion = - 9.5 (T_m - T)

T_m = melting temperature
T = current temperature

ΔG_fusion = 0 at the melting temperature of the component.
ΔG_fusion < 0 below the melting temperature of the component.
ΔG_fusion > 0 above the melting temperature of the component.

In an alloy, if both the liquid and solid solutions are ideal then ΔG_fusion for the alloy can be interpolated between the values for the two components.

Now we can plot the free energy of a regular solid solution from the equation,

ΔG_sol = ΔG_mix + ΔG_fusion

Phase diagrams 1

Free energy curves can be used to determine the most stable state for a system, i.e. the phase or phase mixture with the lowest free energy for a given temperature and composition. Below is a schematic free-energy curve for the solid phase of an alloy.

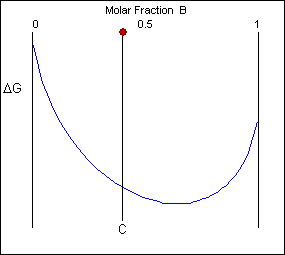
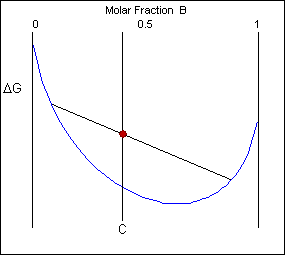
The solid shown could either exist as a mixture or as a homogeneous solution of A and B. The figures below show that an alloy of composition C can exist in different configurations with differing free energies. In the first figure (below) the free energy of unmixed A and B is shown as the diagonal black line. The free energy of this mixture at composition C is shown as a red point.

The system can reduce its free energy by existing as a mixture of two phases

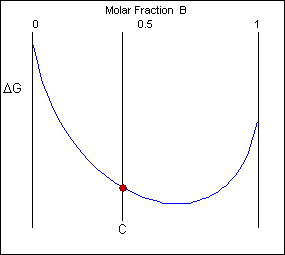
Though the system has reduced its free energy from that of the mixture, the most stable configuration for the system is a solid solution. This allows the free energy of the system to sit on the free energy curve.

For most systems there will be more than one phase and associated free-energy curve to consider. At a given temperature the most stable phase for a system can vary with composition. While the system could consist entirely of the phase which is most stable at a given composition and temperature, if the free energy curves for the two phases cross, the most stable configuration may be a mixture of two phases with compositions differing from that of the overall system. The total free energy of the system in any given two-phase configuration can be found by linking the two phases in question with a straight line on a free-energy plot.

Taking a line that is a common tangent to the two free-energy curves produces the lowest possible free energy for the system as a whole. Where the line meets the free energy curves defines the composition of each phase.

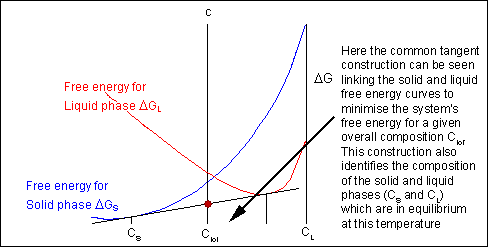
For positions where it is not possible to draw a common tangent between the two free-energy curves the system will sit entirely in the phase with the lowest free energy. The borders between the single- and two-phase regions mark the positions of the solidus and liquidus on the phase diagram.

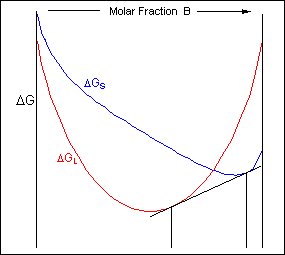
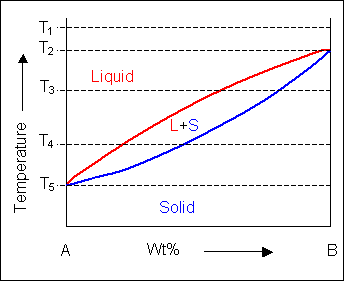
When the temperature is altered the compositions of the solid and liquid in equilibrium change and build up the shape of the solidus and liquidus curves on a phase diagram.

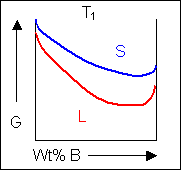
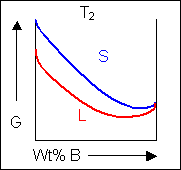
Below, a binary system can be seen along with the free-energy curves for the liquid and solid phases at a range of temperatures shown on the phase diagram.

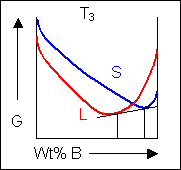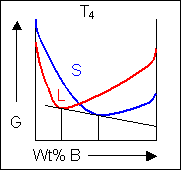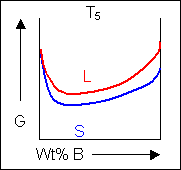Phase diagrams 2 - eutectic reactions

The free-energy curves and phase diagrams discussed in Phase Diagrams 1 were all for systems where the solid exists as a solution at all compositions and temperatures. In most real systems this is not the case. This is due to a positive \( \Delta H_{\rm{mix}} \) caused by unfavourable interactions between unlike neighbour atoms. As the temperature is reduced the \( \Delta H_{\rm{mix}} \) term becomes more significant and the curve turns upward at intermediate compositions, resulting in a curve with two minima and one maximum as described earlier. A common tangent can then be drawn between the two minima showing that the system can reduce its free energy through existing as a mixture of two distinct phases.

The free energy of a system of composition C₀ can be minimised by existing as a mixture of two solid phases of composition C₁ and C₂:

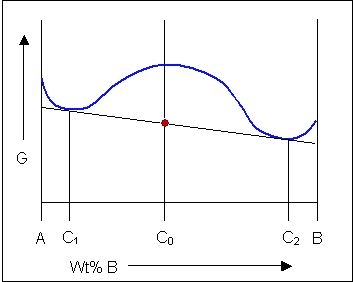
At high temperatures the solid free energy curve will have a single minimum (as the entropy term becomes dominant) and will interact with the liquid free energy curve to produce a two-phase solid + liquid phase field just as it did on the previous page. Alloys in this system will solidify as single-phase solids. However, at low temperatures when the solid free energy curve develops two minima, it is more energetically favourable for the system to separate out into a mixture of two phases.

An example of this can be seen in the Cr-W system:

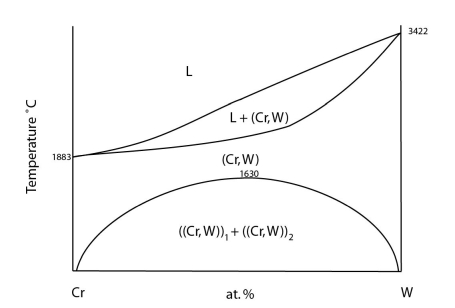
Adapted from https://matdata.asminternational.org/apd/viewPicture.aspx?dbKey=grantami_apd&id=10689446&revision=387514

At high temperatures there is complete solubility in the solid. At low temperatures, a two-phase region develops. The curve separating the single-phase region and the two-phase region is known as the solvus.

In the case above, the liquid free energy curve is high enough energy that it does not interact with the solid free energy curve while it has two minima. This is not the case for many systems.

For a lot of systems, the liquid curve will overlap with the upturned section (between the two minima) of the solid free energy curve for a range of temperatures.

At one specific temperature a common tangent can be drawn for the liquid and solid curves. It is tangent to the solid curve in two places and to the liquid curve in one place. At this temperature, the three phases are in equilibrium.

If the composition of the liquid is intermediate to the two solid phases, the free energy curves for this specific temperature look like this:

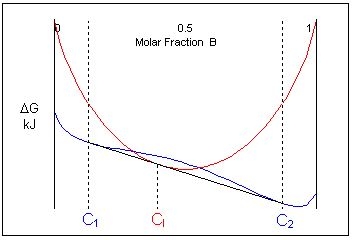
Just below this temperature, at composition C₁, two solid phases are stable and just above this temperature the liquid is the stable phase. This means that an alloy of composition cooling through this temperature will undergo a reaction from two solid phases:

\[ \rm{L \rightleftharpoons \alpha + \beta} \]

This is called a eutectic reaction. The temperature and composition which it occurs at are known as the eutectic temperature and composition. The compositions of the two phases that the liquid transforms into are given by C₁ and C₂.

The eutectic temperature is the lowest melting point in the system.

Eutectic reactions are an example of congruent melting – where a solid melts directly to a liquid without passing through a two-phase solid + liquid region.

Considering the free energy curves for a temperature just above the eutectic temperature, a single common tangent cannot be drawn to link two solid phases and a liquid phase. There is no three-phase equilibrium. Instead, two common tangents are from the liquid curve to the solid curve are drawn instead. This creates two two-phase solid + liquid regions (\( \rm{L + \alpha} \) and \( \rm{L + \beta} \)) and three single-phase regions (\( \rm{L, \alpha} \) and \( \rm{\beta} \)).

At the eutectic temperature, non-eutectic compositions (which still lie within the two-phase solid region) passes into a solid + liquid field. These alloys melt incongruently.

In the phase diagram there is a horizontal (isothermal) line at the eutectic temperature which links the equilibrium compositions of the solids. This is the eutectic invariant reaction line. It is also the solidus for the two-phase solid region of the phase diagram.

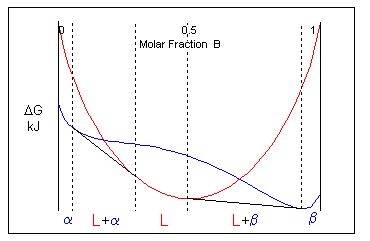
The equilibrium composition of the liquid in the eutectic reaction lies on this invariant reaction line, between the compositions of the solids. At this liquid composition, there is a minimum in the liquidus where it meets the solidus. The point at which they touch is known as the eutectic point.

This is an example of a phase diagram with a eutectic point:

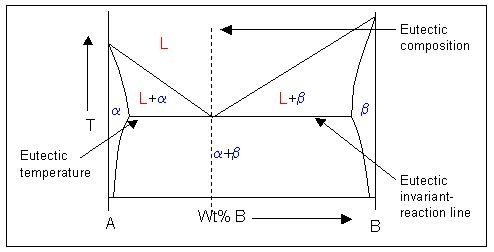
Note: \( \rm{\alpha} \) and \( \rm{\beta} \) are solid phases.

Alloys cooling through the eutectic point (undergoing solidification of \( \rm{\alpha} \) and \( \rm{\beta} \) directly from the liquid) will show 'stripey' microstructure. This is due to the growing A-rich a phase ejecting excess B atoms which can be taken up by neighbouring \( \rm{\beta} \) precipitates (and vice versa). This leads to cooperative growth of the two phases. This causes alternating lamellae of \( \rm{\alpha} \) and \( \rm{\beta}\) to grow, known as the eutectic microstructure.

Some examples of the eutectic microstructure can be seen below:

Cu-Al

From the DoITPoMS micrograph library – micrograph 4

This alloy is of the eutectic composition – 33 wt.% Cu 67 wt.% Al.

The phase diagram for this system is shown below:

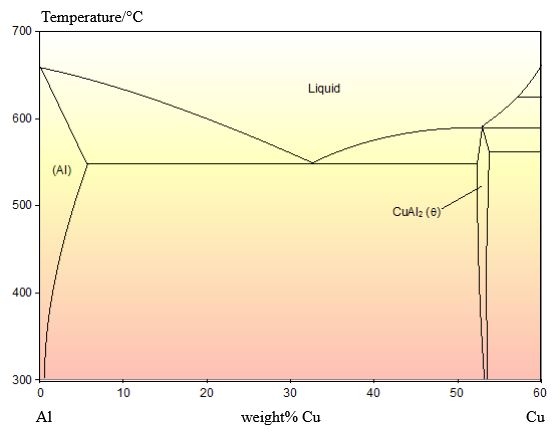
As this alloy is exactly of the eutectic composition, there is no primary solid. The whole microstructure is eutectic intergrowth. The lamellae are very clear in this image.

Cu-P

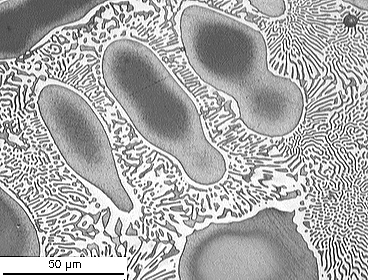
From the DoITPoMS micrograph library – micrograph 698

This alloy has a composition of 4.5 wt.% P balance Cu.

The phase diagram for this system is shown below:

This composition is hypoeutectic so there are primary Cu dendrites. These dendrites are cored (shown by the change in colour from the edge to the centre) due to cooling being too quick to maintain equilibrium. The eutectic reaction occurs once these dendrites have formed, filling the space between them. The stripy eutectic microstructure can be seen clearly in the micrograph.

The eutectic microstructures discussed above are examples of 'regular' or 'normal' eutectics where the growth of the two solid phases is coupled. There is close cooperation between the two solid phases, and they grow at the same rate, sharing an interface with the liquid. Only the plate-like microstructure is discussed above but rod-like microstructure can also arise from normal eutectics. Rod-like microstructures occur when the volume fraction of one phase present is low so an array of rods of the minor phase forms in a matrix of the major phase.

Some eutectics are 'irregular' or 'degenerate'. There is no cooperation between the solid phases in these systems. One phase grows faster than the other and the growth mechanism of this faster growing phase defines the microstructure.

Al-Si

An example of an irregular eutectic can be found in the Al-Si system. The phase diagram is shown below:

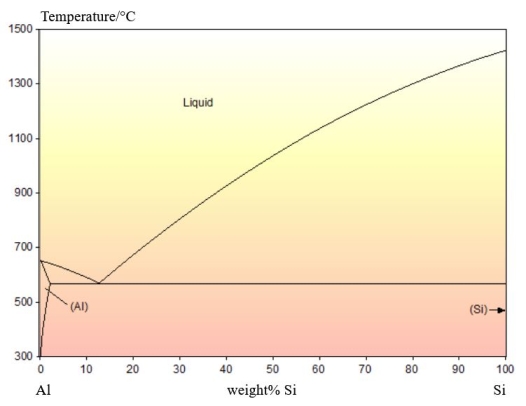
There is no solid solubility of Al in Si. The Si rich phase that forms is pure Si.

Pure Si is covalently bonded. This results in it only growing along well-defined 'fast growth' directions. The Al phase does not have a fast growth direction so grows slower than the Si phase.

The microstructure of Si-Al alloys is dominated by the growth of Si which forms as irregular plates:

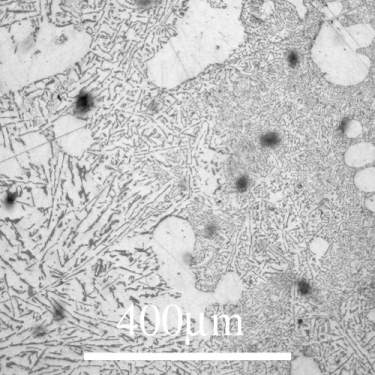
From the DoITPoMS micrograph library – micrograph 698

The above alloy is 12 wt.% Si balance Al, which is the eutectic composition for the system. Some Al primary solid can be seen in the micrograph however which is not expected in a eutectic composition alloy. This is due to the coupling (or lack thereof) of the phases upon cooling.

This microstructure is very brittle due to the large silicon flakes which can act as stress concentrators, so this alloy is rarely used in this state. Often the alloy is cooled rapidly which causes the silicon to grow as fibrous precipitates instead or a small amount of another element (commonly Na or Sr) is added causing the morphology of the silicon precipitates to change (the reason for this is not fully understood).

Other irregular eutectic microstructures have been observed. For example: spiral, cellular, globular and acicular.

Phase diagrams 3 - peritectic reactions

The peritectic reaction, like the eutectic reaction, arises from a three-phase equilibrium.

Similar to the eutectic reaction, the peritectic reaction involves a liquid phase and two solid phases, however in the peritectic reaction the composition of the liquid phase lies outside of the two solid phases (e.g., more A or B rich than both solid phases).

The three phases are in equilibrium at the peritectic temperature. The free energy curves for this temperature are shown below:

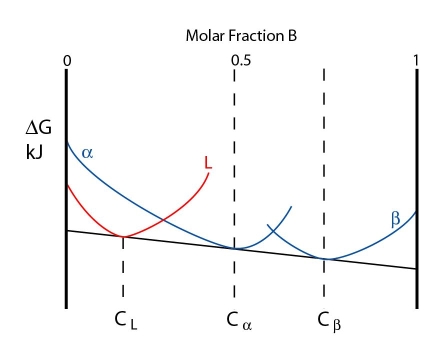
Note: In this case the free energy curves for \( \rm{\alpha} \) and \( \rm{\beta} \) are represented as separate curves, rather than a single continuous curve.

At this temperature the three phases are in equilibrium. However, just below the peritectic temperature the alpha and beta phases lower in energy while the liquid curve is raised. A common tangent can no longer be drawn for all three phases. The free energy curves are shown below:

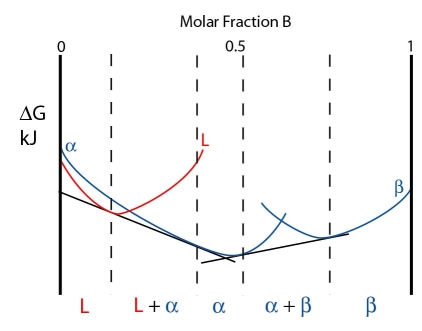
As a single common tangent for all three phases is impossible, two common tangents are drawn instead: one from the \( \rm{\alpha} \) curve to the liquid curve and one from the \( \rm{\alpha} \) curve to the \( \rm{\beta} \) curve. The system minimises its free energy by having two two-phase regions (\( \rm{L + \alpha} \) and \( \rm{L + \beta} \)).

Above the peritectic temperature, the liquid free energy curve lowers in energy while the \( \rm{\alpha} \) and \( \rm{\beta} \) curves rise in energy. The \( \rm{\alpha} \) curve rises more rapidly, however.

The free energy curves for a temperature just above the peritectic temperature are shown below:

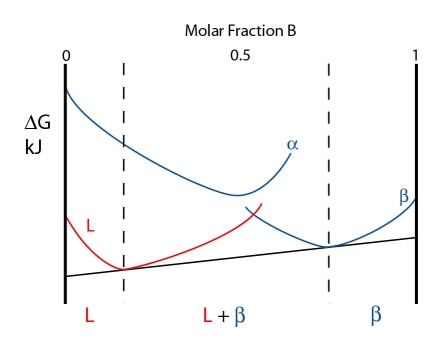
As the \( \rm{\alpha} \) curve is so high energy, a common tangent cannot be drawn from it. Instead, the system minimises its free energy by forming a \( \rm{L + \beta} \). The composition of the \( \rm{L + \beta} \) phase are given by the contacts of the common tangent with the free energy curves.

For an alloy of composition \( \rm{C_{\alpha}} \) (the composition of the a phase at the peritectic temperature) the equilibrium phases above the peritectic temperature are \( \rm{L} \) and \( \rm{\beta} \). The equilibrium phase for this alloy just below the peritectic temperature is \( \alpha \).

This means that alloys at this composition \( \rm{C_{\alpha}} \) cooling through the peritectic temperature undergo a reaction:

\[ \rm{L + \beta \rightleftharpoons \alpha} \]

The liquid reacts with the solid \( \rm{\beta} \) to form solid \( \rm{\alpha} \). This is the peritectic reaction. The composition it occurs at (\( \rm{C_{\alpha}} \)) is the peritectic composition. The composition of the liquid and \( \rm{\beta} \) are given by C_L and \( \rm{C_{\beta}} \) respectively.

In this system there is a horizontal line at the peritectic temperature. This is the peritectic invariant reaction line. Part of this line is the solidus for the two-phase solid region.

One end of this line gives the equilibrium composition in the peritectic reaction. The other end gives the composition of the \( \rm{\beta} \) phase. The composition of the a phase lies at an intermediate point on the line. At this composition the a phase field has a maximum where it meets the solidus. This is the peritectic point.

From the energy curves it can be seen that in this system the \( \rm{L + \beta} \) phase field only exists above the peritectic temperature while the \( \rm{L + \alpha} \) phase field only exists below the peritectic temperature.

This is an example of a phase diagram with a peritectic point:

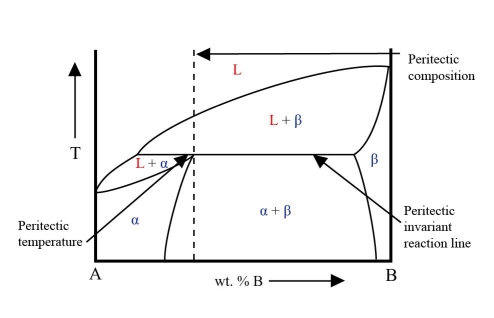
During the peritectic reaction, as the liquid reacts with \( \rm{\beta} \), \( \rm{\alpha} \) is precipitated heterogeneously on the pre-existing \( \rm{\beta} \) precipitates. This coating of \( \rm{\alpha} \) on \( \rm{\beta} \) precipitates stops contact between the liquid and \( \rm{\beta} \) preventing reaction from going to completion.

Very slow cooling rates are required to allow a peritectic reaction to go to completion for this reason. As a result, the microstructure of most alloys going through a peritectic reaction show primary precipitates covered in secondary solids in a matrix of a completely different composition to the bulk composition.

An example of the peritectic reaction failing to go to completion can be seen in the Cu-Sn system:

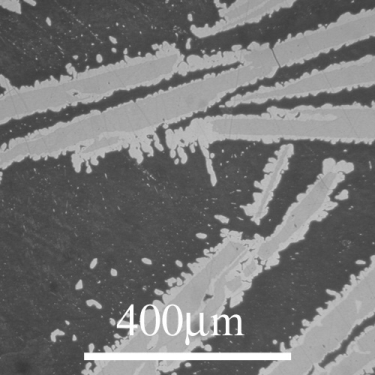
From the DoITPoMS micrograph library – micrograph 12. This alloy is 21 wt.% Cu 79 wt. % Sn.

The phase diagram is shown below:

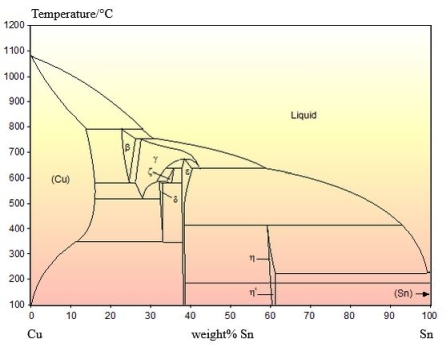
The peritectic reaction that this alloy cools through is the reaction. In the micrograph, primary \( \varepsilon \) solids can be seen as the darker grey cores of the precipitates. The lighter grey coating on these primary solids is \( \eta \). As the \( \eta \) grows on the \( \varepsilon \) solids, the liquid loses contact with the \( \varepsilon \) solids and the peritectic reaction ceases.

This leaves the remaining liquid more Sn rich than would be expected at equilibrium. There is a eutectic reaction at around 230°C very close to the Sn side of the phase diagram. The remaining liquid cools through this reaction and solidifies nearly entirely as solid Sn. This makes up the matrix that the \( \varepsilon \) and \( \eta \) sit in. This is micrograph is not in equilibrium but systems cooling through peritectic reactions rarely are.

Determining Reaction Type

In a reaction the two 'outside' phases (those with the most extreme compositions – the richest in A and the richest in B) must react to form the 'inner' phase (that with the intermediate composition to the other two). This is required by conservation of matter.

In the case of a eutectic reaction, \( \rm{\alpha} \) and the liquid phase are both richer in A than the \( \rm{\beta} \) phase. If \( \rm{\alpha} \) reacted with the liquid, the resulting mixture cannot be as rich in B as \( \rm{\beta} \) is. Therefore, \( \rm{\alpha} \) and the liquid cannot react to form \( \rm{\beta} \) - there are simply not enough B atoms in the \( \rm{\alpha} \) or liquid phase to allow it.

The reaction occurring in a phase diagram can be determined by identifying the invariant reaction line and finding the 'outside' and inside phases. The outside phases go to form the inside phase. From this, the form of the reaction can be written. By observing the phases present above and below the invariant temperature, the direction of the reaction with decreasing temperature (as it is conventionally written) can be found.

The Gibbs phase rule

The Gibbs phase rule links the number of phases, number of components and number of degrees of freedom in a system.

A phase (p) can be defined as the homogenous regions of a system. Phases are separated from each other by bounding surfaces. A system which contains one phase when in equilibrium is known as homogenous. If a system has more than one phase present in equilibrium, then it is known as heterogenous. In heterogeneous systems, it is (at least in theory) possible to mechanically separate the various phases from each other.

The number of components in a system (c) is analogous to a set of basis vectors for a space. It is the smallest number of independent variables required to completely describe the composition of all of the phases in the system. In a unary system (where a material of a single composition is considered) the number of components is one. In a binary system (like where two metals are mixed together to form an alloy) the number of components is two and the composition is represented as a line going from 100% of one component to 100% of the other component.

The number of degrees of freedom of an equilibrium state (f) is the number of variables (composition variable, temperature or pressure) that must be specified to define the state of a system.

Derivation of the Phase Rule

The composition of each phase in a system is defined by c−1 variables. By specifying the proportion of c−1 components, the proportion of the last one is defined (as the fraction of each proportion must sum to 1). In a binary system, stating that there is X% of one component implies that there is (1−X)% of the other component.

There are p phases in a system. It follows that the number of compositional variables in the system is then p(c−1) - the number of phases multiplied by the number of variables per phase.

The total number of variables in the system is given by p(c−1)+2 – the number of compositional variables plus a variable each for pressure and temperature.

The number of degrees of freedom in the system is given by the difference of the total number of variables in the system and the number of variables that are set by specifying that the system is in equilibrium.

A requirement for a system to be in equilibrium is that the chemical potential of each component is the same in each phase.

There are p−1 independent equations describing each component in each phase. By specifying the chemical potential are equal to each other in all combinations of phases but one, the fact that they are equal in this final pair of phases is implied. It is a dependent equation (can be constructed from the others) so is not considered.

When the system is defined to be in equilibrium, c(p−1) variables are specified (p−1 for each component, multiplied by the number of components).

The number of degrees of freedom is therefore:

Degrees of freedom = total variables − specified variables

\[ f = p(c-1)+2-c(p-1) \]

Simplifying:

\[ f + p = c + 2 \]

This is known as the Gibbs phase rule.

Applying the Gibbs Phase Rule

Unary Systems

In a unary system, the number of components is one, c = 1.

The total number of degrees of freedom and phases possible is therefore three.

There can be:

• One phase and two degrees of freedom
• Two phases and one degree of freedom
• Three phases and no degrees of freedom

There cannot be a case where no phases exist.

Consider the pressure-temperature phase diagram for water:

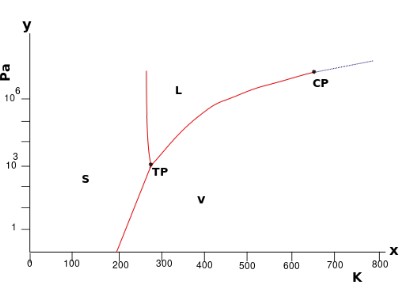
By Eurico Zimbres - Own work, CC BY-SA 2.5, https://commons.wikimedia.org/w/index.php?curid=1173910

It is a unary phase diagram. There is one component – water.

In the single-phase regions (solid, liquid and vapour) there are two degrees of freedom. The equilibrium is bivariant. The system can lie on any point in this region so both the temperature and pressure must be specified to define the state of the system.

Both the temperature and the pressure can be altered without moving out of the single-phase region.

Two phase equilibria are represented by the lines bounding the single-phase regions. These lines are pressure – temperature conditions where two phases can coexist. On these lines, there is one degree of freedom. The equilibrium is univariant.

By specifying the temperature or pressure, the other variable is constrained (as the system must lie on the bivariant line). Only one variable is needed to define the system.

Three phase equilibria (which exists at the triple point here) have no degrees of freedom. They are invariant equilibria. The invariant point has a set temperature and pressure so requires no variables to be specified.

By stating that the system is in an invariant equilibrium, the temperature and pressure are implied.

Binary Systems

In a binary system the number of components is two, c = 2. The total number of degrees of freedom and phases is four.

As binary systems are often considered on composition-temperature phase diagrams, pressure is fixed. This removes a degree of freedom (as changing the pressure requires moving off the plane of the binary phase diagram).

For a constant pressure (or equally a constant temperature), the total number of phases and degrees of freedom is three.

There can be:

• One phase and two degrees of freedom
• Two phases and one degree of freedom
• Three phases and no degrees of freedom<

Consider a simple binary eutectic phase diagram:

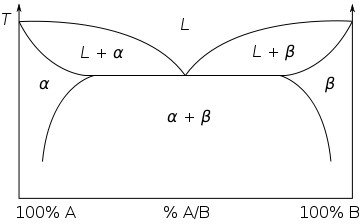
By Eutektikum.gif: Dr. Báder Imrederivative work: Michbich (talk) - Eutektikum.gif, CC BY-SA 3.0, https://commons.wikimedia.org/w/index.php?curid=8394880

Single phase regions are areas where the alloy can lie on any point in the region.

Single phase regions have two degrees of freedom. These are bivariant equilibria. Both a temperature and a composition must be specified to define the state of the system.

Two phase regions can be thought of as a stack of isothermal tie lines. Each end of the tie line gives the composition of the phases at that temperature. The bounding curves of the two-phase regions are mapped out by the ends of these tie lines.

Two phase regions are areas, and the alloy can lie on any tie line within the area.

Two phase regions only have one degree of freedom, they are univariant equilibria.

By specifying the temperature, the relevant tie line can be identified and the compositions of the two phases determined, defining the state of the system.

Similarly, by specifying the composition of one of the two phases, the relevant tie line can be identified and from it the temperature and the composition of the other phase determined. By specifying that there are two phases in equilibrium and one variable, the system is defined.

Three phase regions have no degrees of freedom. They are invariant equilibria. They are points on the phase diagram with a fixed temperature and composition. The invariant point in this phase diagram is the eutectic point where alpha, beta and the liquid are the three phases in equilibrium. The temperature and composition cannot be changed without moving off of the invariant point.

Reactions are often associated with invariant points as heating or cooling through an invariant point can cause a dramatic change in the phases present. Some examples of invariant reactions in binary systems are:

Eutectic      \( \rm{L \rightleftharpoons \alpha + \beta} \)  seen in Ag-Cu, Al-Si, Sn-Bi and Fe-C systems

Peritectic    \( \rm{L + \alpha \rightleftharpoons \beta} \)  seen in Cu-Zn, Cu-Sn and Fe-C systems

Eutectoid    \( \rm{\alpha \rightleftharpoons \beta + \gamma} \)  seen in Fe-C system

Peritectoid  \( \rm{\alpha + \beta \rightleftharpoons \gamma} \)  seen in Al-Cu system

Monotectic  \( \rm{L_1 \rightleftharpoons L_2 + \alpha} \) seen in Cu-Pb system

Metatectic   \( \rm{\alpha \rightleftharpoons \beta + L} \)  seen in Ag-Li system

Syntactic     \( \rm{L_1 + L_2 \rightleftharpoons \alpha} \) seen in K-Zn system

Limitations of the Gibbs Phase Rule

The Gibbs phase rule determines what combination of phases and degrees of freedom are possible in a system. It cannot inform on which kinds of equilibria are present in each system, simply the nature of those equilibria if they were present.

Interpretation of cooling curves

The melting temperature of any pure material (a one-component system) at constant pressure is a single unique temperature. The liquid and solid phases exist together in equilibrium only at this temperature. When cooled, the temperature of the molten material will steadily decrease until the melting point is reached.

At this point the material will start to crystallise, leading to the evolution of latent heat at the solid liquid interface, maintaining a constant temperature across the material. Once solidification is complete, steady cooling resumes. The arrest in cooling during solidification allows the melting point of the material to be identified on a time-temperature curve.

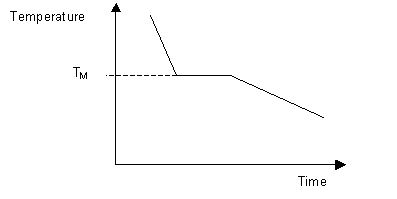
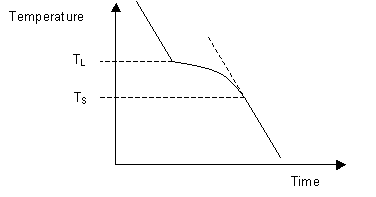
Most systems consisting of two or more components exhibit a temperature range over which the solid and liquid phases are in equilibrium. Instead of a single melting temperature, the system now has two different temperatures, the liquidus temperature and the solidus temperature which are needed to describe the change from liquid to solid.

The liquidus temperature is the temperature above which the system is entirely liquid, and the solidus is the temperature below which the system is completely solid. Between these two points the liquid and solid phases are in equilibrium. When the liquidus temperature is reached, solidification begins and there is a reduction in cooling rate caused by latent heat evolution and a consequent reduction in the gradient of the cooling curve.

Upon the completion of solidification the cooling rate alters again allowing the temperature of the solidus to be determined. As can be seen on the diagram below, these changes in gradient allow the liquidus temperature T_L, and the solidus temperature T_S to be identified.

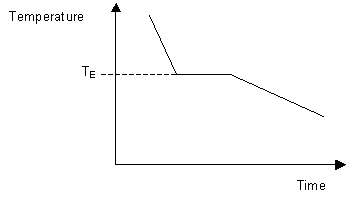
When cooling a material of eutectic composition, solidification of the whole sample takes place at a single temperature. This results in a cooling curve similar in shape to that of a single-component system with the system solidifying at its eutectic temperature.

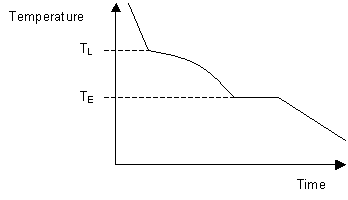
When solidifying hypoeutectic or hypereutectic alloys, the first solid to form is a single phase which has a composition different to that of the liquid. This causes the liquid composition to approach that of the eutectic as cooling occurs. Once the liquid reaches the eutectic temperature it will have the eutectic composition and will freeze at that temperature to form a solid eutectic mixture of two phases.

Formation of the eutectic causes the system to cease cooling until solidification is complete. The resulting cooling curve shows the two stages of solidification with a section of reduced gradient where a single phase is solidifying and a plateau where eutectic is solidifying.

By taking a series of cooling curves for the same system over a range of compositions the liquidus and solidus temperatures for each composition can be determined allowing the solidus and liquidus to be mapped to determine the phase diagram.

Below are cooling curves for the same system recorded for different compositions and then displaced along the time axis. The red regions indicate where the material is liquid, the blue regions indicate where the material is solid and the green regions indicate where the solid and liquid phases are in equilibrium.

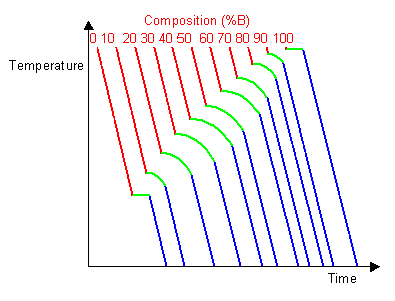
By removing the time axis from the curves and replacing it with composition, the cooling curves indicate the temperatures of the solidus and liquidus for a given composition.

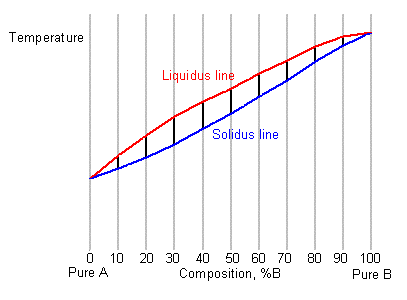
This allows the solidus and liquidus to be plotted to produce the phase diagram:

Experiment and results

The simplest way to construct a phase diagram is by plotting the temperature of a liquid against time as it cools and turns into a solid. As discussed in Interpretation of cooling curves, the solidus and liquidus can be seen on the graphs as the points where the cooling is retarded by the emission of latent heat.

Method

An experiment can be performed to get a rough idea of a phase diagram by recording cooling curves for alloys of two metals, in various compositions. The alloy chosen for this example is bismuth-tin, both of which metals have low melting points, and so can be heated and cooled more quickly and easily in the lab. So that the experiment could be performed in a reasonable time, 11 compositions were used, from pure bismuth to pure tin in steps of 10%. All the compositions were measured in weight percent.

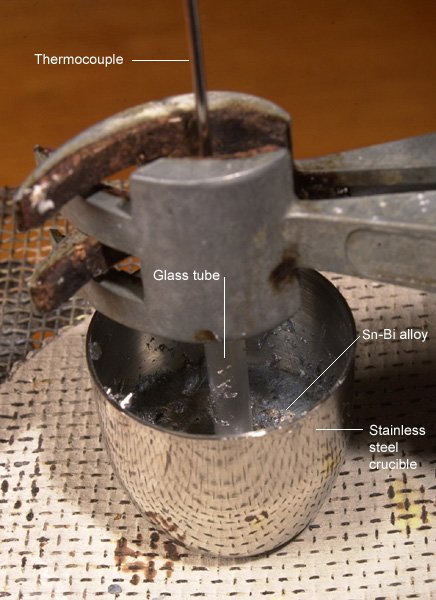Heating apparatus	Crucible
(Click on image to view a larger version)

The apparatus pictured was set up, with the maximum temperature to be attained of around 300°C. The sample in the crucible cooled, and the temperature was recorded at regular intervals. In the case of the results produced here, readings were made every two seconds, using a computer. However, it is adequate to take readings every 15 seconds manually.

Results

The procedure was repeated for all 11 compositions, and the following results recorded:

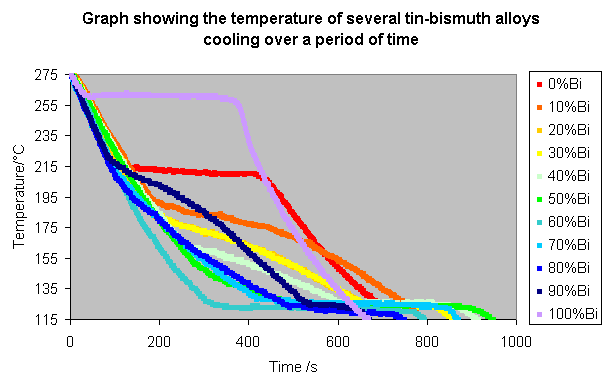
These lines can be made clearer by spacing them along the time axis, so that the alloys with a higher bismuth content appear further to the right, as shown below.

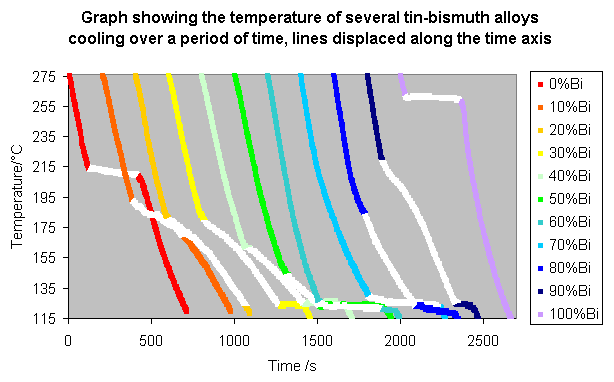
From these graphs it is possible to pick out the changes in gradient which allow a simplified phase diagram to be drawn.

On the diagram with the displaced time-temperature plots, the changed gradients, i.e. the parts where some of the liquid is solidifying, are coloured white. These show the top of the liquidus and the bottom of the solidus.

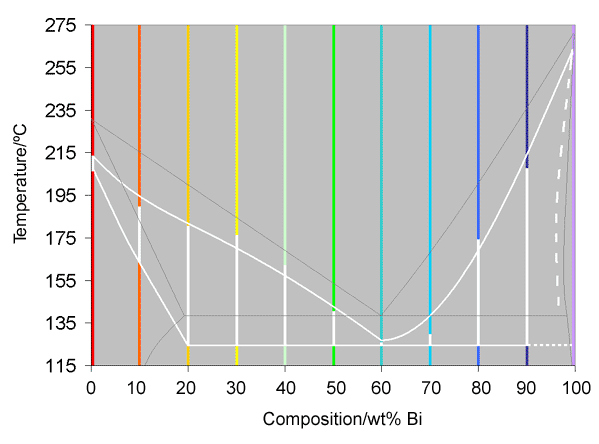
If these curves are now straightened out, and the colours kept, with the white representing the solidification, it is possible to construct a basic phase diagram, as was shown for an isomorphous system in Interpretation of cooling curves. This is shown below, where the white line is part of the phase diagram, constructed from the cooling curves, and the thin grey line is the actual phase diagram, found from many experiments over a long period of time.

Analysis

It can be seen from the diagram above that the recorded phase diagram is roughly 15°C lower than it should be, and that some of the measurements of the liquidus are not in the expected places. The lowering of the diagram is due to the thermocouple being contained in a glass rod, rather than actually touching the alloy. The occasional unexpected liquidus temperatures are probably due to the compositions being slightly inaccurate.

It is also worth noting that in this projected phase diagram, it was not possible to draw in a proper solidus on the right hand side, as none of the compositions near pure bismuth showed evidence of a solidus. As such, a dotted line has been plotted as an estimate of where it would go.

The microstructure of the alloy changes with composition. This can be seen in scanning electron microscope (SEM) images taken from each of the compositions used in the experiment above.

Interactive Sn-Bi phase diagram and SEM images

The lever rule

If an alloy consists of more than one phase, the amount of each phase present can be found by applying the lever rule to the phase diagram.

The lever rule can be explained by considering a simple balance. The composition of the alloy is represented by the fulcrum, and the compositions of the two phases by the ends of a bar. The proportions of the phases present are determined by the weights needed to balance the system.

So,

fraction of phase 1 = (C₂ - C) / (C₂ - C₁)

and,

fraction of phase 2 = (C - C₁) / (C₂ - C₁).

Lever rule applied to a binary system

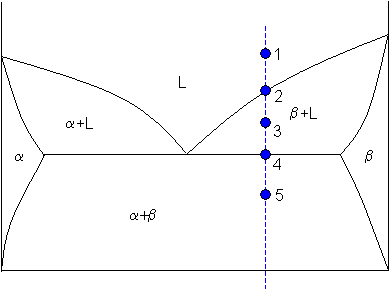
Point 1

At point 1 the alloy is completely liquid, with a composition C. Let

C = 65 weight% B.

Point 2

At point 2 the alloy has cooled as far as the liquidus, and solid phase β starts to form. Phase β first forms with a composition of 96 weight% B. The green dashed line below is an example of a tie-line. A tie-line is a horizontal (i.e., constant-temperature) line through the chosen point, which intersects the phase boundary lines on either side.

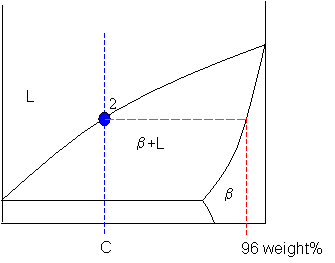
Point 3

A tie-line is drawn through the point, and the lever rule is applied to identify the proportions of phases present.

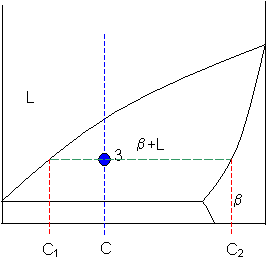
Intersection of the lines gives compositions C₁ and C₂ as shown.

Let

C₁ = 58 weight% B

and

C₂ = 92 weight% B

So,

fraction of solid β = (65 - 58) / (92 - 58) = 20 weight%

and

fraction of liquid = (92 - 65) / (92 - 58) = 80 weight%

Point 4

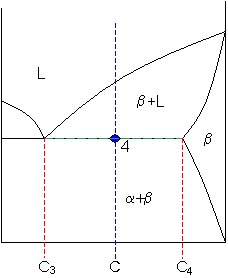
Let

C₃ = 48 weight% B

and

C₄ = 87 weight% B

So

fraction of solid β = (65 - 48) / (87 - 48) = 44 weight%.

As the alloy is cooled, more solid β phase forms.

At point 4, the remainder of the liquid becomes a eutectic phase of α+β and

fraction of eutectic = 56 weight%

Point 5

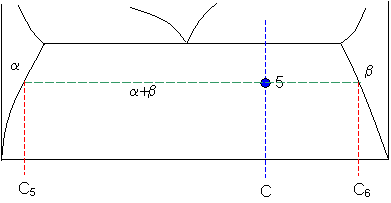
Let

C₅ = 9 weight% B

and

C₆ = 91 weight% B

So

fraction of solid β = (65 - 9) / (91 - 9) = 68 weight%

and

fraction of solid α = (91 - 65) / (91 - 9) = 32 weight%.

Modern uses

Phase diagrams are not just an abstract construction - they have applications in the real world, in deciding which compositions to use.

A major use of eutectics, or near eutectics is in solder. In plumbing, solder is used to join copper pipes together, producing a waterproof seal. For many years a lead-tin alloy has been used, as this has a low melting point, especially at eutectic. However, although a low melting point is sought, it is useful to be able to move the pipes around slightly when they are in place and the solder is solidifying. This means that a eutectic should not be used, as although it has the lowest melting point for the alloy system, it will all solidify at once, leaving little room for error. Instead it is useful to use an alloy whose composition deviates slightly from that of the eutectic, so that the solidification will take longer, making the solder easier to use, despite the higher temperatures, and so resulting in a better join.

Electrical solder uses a similar alloy to join parts of an electronic circuit together. In the case of a standard electrical solder, the eutectic is used, as high temperatures are to be avoided, and it is useful for the solder to solidify all at once.

In more modern soldering applications, such as a ball grid array which joins the chip to some circuit boards, the eutectic is still used. However, there are also situations where a slightly off-eutectic can be used. If there are several processing steps, it is useful to start off with a higher melting point alloy, and only use the eutectic in the final soldering stage. This allows the previous solders to stay in place when the heating takes place on the later stages.

Modern solders have moved away from lead-based alloys, because of environmental considerations, and been replaced with new alloys. In the case of plumbing, there is a tendency to use plastic piping instead of copper piping, so there is less need for solder in this industry. In the electronics industry, lead-tin is being replaced by copper-, tin-, and silver-based systems, which have less environmental problems, but can still be used to create low melting points and flexibility which the lead-tin systems provide. Lots of the modern research on solders relates to alternative systems, and characterising them for use. Part of this characterisation involves the production of phase diagrams to allow good choice of composition for the right properties.

In recent times, it has become necessary to mix several elements in order to improve properties of the materials. It is therefore useful to create phase diagrams which involve three elements, called "ternary" diagrams. These are more complicated than two element "binary" phase diagrams, but allow improved optimisation, and hence can produce better results.

Further considerations

Other methods for constructing phase diagrams

Although the easiest way to investigate phase transformations is by the use of time temperature cooling curves, there are many ways to investigate these changes. This is most helpful when observing solid to solid phase changes, as these take longer to reach equilibrium, and so the cooling of a material must take place at a slower rate in order to get accurate results. Unfortunately, as the rate of cooling decreases, it gets harder to detect the latent heat being released, and so other methods must be sought.

The thinking behind most of the methods is the same; as the material changes phase, there will be changes in its physical properties. As such, the phase change can be detected by observing a property as the temperature is reduced. Examples of this are density, electrical or thermal resistance, optical properties, Young's modulus and damping efficiency. Another frequently used measure is that of interplanar spacings in the crystalline phases, which can be measured using X-ray diffractometry.

These techniques have been used to map out phase diagrams, but they are always time consuming, especially during the solid transformations.

Dangers in interpretation of phase diagrams

An essential point to remember in phase diagrams is that during normal or fast cooling, results may not be as expected in the diagram. Both the theory and the experiments to construct phase diagrams rely on the assumption that the system is in equilibrium, which is rarely the case, as this only occurs properly when the system is cooled very slowly. In order to reach full equilibrium, the solute in the solid phases must stay completely uniform throughout the cooling. However, in most systems, if the system is not cooled quickly, the phase diagram will give fairly accurate results. In addition, near the eutectic, the results become even closer to the phase diagram, as the liquid solidifies at nearly the same time.

The non equilibrium conditions can sometimes be of benefit however, as microstructures at higher temperatures in a phase diagrams may sometimes be preserved to lower temperatures by fast cooling, i.e. quenching, or unstable microstructures may occur during fast cooling which can be useful when hardening an alloy.

Summary

In this package, the theoretical background to phase diagrams has been shown, as well as a method for constructing part of a diagram. Explanation has been given of how to use a phase diagram, and how it applies to real systems, and to understanding solidification.

It should now be appreciated that phase diagrams are a valuable resource in predicting behaviour of alloys during solidification, and the microstructures which will be produced.

However, there should also be an understanding that in normal cooling conditions although phase diagrams are generally fairly accurate, they are not always exact, and if diffusion is slow, there may be unexpected results.

Questions

Quick questions

You should be able to answer these questions without too much difficulty after studying this TLP. If not, then you should go through it again!

1. What is the effect on the shape of the free-energy curve for a solution if its interaction parameter is positive?

   	a	Produces a curve which has one minimum
   	b	Produces a curve with no minimum and one maximum
   	c	Produces a curve which contains a maximum at low T
   	d	Produces a curve which contains a maximum at high T

2. In terms of interatomic bonding, what does a negative interaction parameter represent?

   	a	That A-A and B-B bonds are more favoured
   	b	That A-B bonds are more favoured
   	c	That A-A bonds are more favourable than B-B bonds

3. Cooling curve for a binary system:

   

   	a	One phase
   	b	Two phases
   	c	Three phases
   	d	Four phases

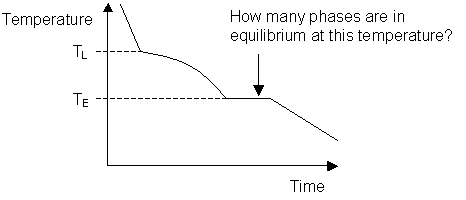
4. What is a hypoeutectic alloy?

   	a	An alloy which has solute content greater than that of the eutectic.
   	b	An alloy which has a solute content lower than that of the eutectic.
   	c	An alloy whose solute content is such that it contains no eutectic.
   	d	An alloy whose final microstructure is wholly eutectic.

5. Look at the following Al-Si phase diagram. (Place the mouse over the diagram to determine the temperature and composition at any point.)

   For an Al 5 wt% Si alloy what will be the composition of the solid in equilibrium with the liquid at 600°C?

   	a	1 wt%
   	b	2 wt%
   	c	9 wt%
   	d	13 wt%

6. Look at the following Cu-Al phase diagram. (Place the mouse over the diagram to determine the temperature and composition at any point.)

   What will be the relative weight fraction of CuAl₂ (θ) in a Al 15wt% Cu alloy at its eutectic temperature?

   	a	66.6%
   	b	33.3%
   	c	50%
   	d	75%

Deeper questions

The following questions require some thought and reaching the answer may require you to think beyond the contents of this TLP.

7. Using the following data, calculate the volume fraction of the beta phase and eutectic at the eutectic temperature, for an alloy of composition 75 wt% Ag. Assume equilibrium conditions.

   At eutectic temp:

   eutectic composition = 71.9 wt% Ag
   maximum solid solubility of Cu in Ag = 8.8 wt% Cu
   density Ag = 10 490 kg/m³
   density Cu = 8 920 kg/m³
   

8. Composition vs temperature phase diagrams exist for the combinations of three elements A, B and C (i.e. the three phase diagrams A-B, A-C and B-C). How might they be arranged in three-dimensional space to construct a "ternary" phase diagram for a system containing A, B and C?

Open-ended questions

The following questions are not provided with answers, but intended to provide food for thought and points for further discussion with other students and teachers.

9. Under what conditions could the compositions of the phases present differ from that predicted in the phase diagram?

Going further

Books

• Porter, D. A. and Easterling K, Phase Transformations in Metals and Alloys, 2nd edition, Routledge, 1992.
• Smallman, R. E, Modern Physical Metallurgy, Butterworth, 1985.
• John, V, Understanding Phase Diagrams, Macmillan,1974.

Websites

• Alloys and their phase diagrams - including examples of Binary Isomorphous Alloy Systems
  An Adobe Acrobat file authored by Professor P.J.M. Monteiro at the University of California, Berkeley.
• Solidification
  A MATTER module with storyboard by Professor Bill Clyne providing an in-depth look at processes involved in solidification.

---

Stirling's approximation

Stirling's approximation is:

ln N! = NlnN - N, for large N

The entropy,

S = k lnw

where w is the number of possible configurations for a system.

For a mechanical mixture w = 1 as the only arrangement is A atoms on A sites and B atoms on B sites.

For a solid solution of A and B containing x_AN A atoms and x_BN B atoms the value of w is calculated as follows

	N!
	{xAN}!{(1 - xA)N}!

Assuming that the thermal entropy of the system remains unchanged when A and B go into solution

	ΔSmix	=	k ln   N!   -k ln1 {xAN}!{(1 - xA)N}!
		=	k [ln N! - ln {xAN}! - ln {(1 - xA)N}!]
		=	k [ N ln N - N - xAN ln xAN + xAN - (1 - xA)N ln (1 - xA)N + (1 - xA)N]
		=	kN [ln N - 1 - xA ln xAN + xA - (1 - xA) ln (1 - xA)N + (1 - xA)]
		=	kN [ln N - xA ln {xAN} - (1 - xA) ln {(1 - xA)N}]
		=	kN [ln N - xA ln xA - xA ln N - (1 - xA) ln (1 - xA) - ln N + xA ln N
		=	kN [- xA ln xA - (1 - xA) ln (1 - xA)]
		=	kN [- xA ln xA - xB ln xB]

---

Academic consultant: Lindsay Greer (University of Cambridge)
Content development: Matt Charles, Jacqui Capes and Andrew Cockburn
Photography and video: Brian Barber and Carol Best
Web development: Dave Hudson

This TLP was prepared when DoITPoMS was funded by the Higher Education Funding Council for England (HEFCE) and the Department for Employment and Learning (DEL) under the Fund for the Development of Teaching and Learning (FDTL).

Additional support for the development of this TLP came from the Armourers and Brasiers' Company and Alcan.